Convex hull - sweep line

https://www.topcoder.com/community/competitive-programming/tutorials/line-sweep-algorithms/

The convex hull of a set of points is the smallest convex polygon that surrounds the entire set, and has a number of practical applications. An efficient method that is often used in challenges is the Graham scan [2], which requires a sort by angle. This isn’t as easy as it looks at first, since computing the actual angles is expensive and introduces problems with numeric error. A simpler yet equally efficient algorithm is due to Andrew [1], and requires only a sort by X for a line sweep (although Andrew’s original paper sorts by Y and has a few optimizations I won’t discuss here).

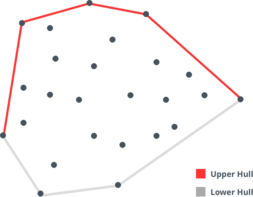
Andrew’s algorithm splits the convex hull into two parts, the upper and lower hull. Usually these meet at the ends, but if more than one points has minimal (or maximal) X coordinate, then they are joined by a vertical line segment. We’ll describe just how to construct the upper hull; the lower hull can be constructed in similar fashion, and in fact can be built in the same loop.

To build the upper hull, we start with the point with minimal X coordinate, breaking ties by taking the largest Y coordinate. After this, points are added in order of X coordinate (always taking the largest Y value when multiple points have the same X value). Of course, sometimes this will cause the hull to become concave instead of convex:

The black path shows the current hull. After adding point 7, we check whether the last triangle (5, 6, 7) is convex. In this case it isn’t, so we delete the second-last point, namely 6. The process is repeated until a convex triangle is found. In this case we also examine (4, 5, 7) and delete 5 before examining (1, 4, 7) and finding that it is convex, before proceeding to the next point. This is essentially the same procedure that is used in the Graham scan, but proceeding in order of X coordinate rather than in order of the angle made with the starting point. It may at first appear that this process is O(N2) because of the inner backtracking loop, but since no point can be deleted more than once it is in fact O(N). The algorithm over-all is O(N log N), because the points must initially be sorted by X coordinate.

https://www.hackerearth.com/practice/math/geometry/line-sweep-technique/tutorial/
Let S be a set of points. Then, convex hull is the smallest convex polygon which covers all the points of S.
There exists an efficient algorithm for convex hull (Graham Scan) but here we discuss the same idea except for we sort on the basis of x coordinates instead of angle.
The pseudo code for the algorithm is:

Sort the points of P by x-coordinate (in case of a tie, sort by y-coordinate).

Initialize U and L as empty lists.
The lists will hold the vertices of upper and lower hulls respectively.

for i = 1, 2, ..., n:
    while L contains at least two points and the sequence of last two points
            of L and the point P[i] does not make a counter-clockwise turn:
        remove the last point from L
    append P[i] to L

for i = n, n-1, ..., 1:
    while U contains at least two points and the sequence of last two points
            of U and the point P[i] does not make a counter-clockwise turn:
        remove the last point from U
    append P[i] to U

Remove the last point of each list (it's the same as the first point of the other list).
Concatenate L and U to obtain the convex hull of P.
Points in the result will be listed in counter-clockwise order.

C++ implementation of the above algorithm is as follows:

struct Point {
        double x, y;
};
bool compare(Point a,Point b)
{
        return a.x<b.x || (a.x==b.x && a.y<b.y);
}
//Returns positive value if B lies to the left of OA, negative if B lies to the right of OA, 0 if collinear
double cross(const Point &O, const Point &A, const Point &B)
{
        return (A.x - O.x) * (B.y - O.y) - (A.y - O.y) * (B.x - O.x);
}
//Returns a list of points on the convex hull
vector<Point> convex_hull(vector<Point> P)
{
        int n = P.size(), k = 0;
        vector<Point> H(2*n);
        sort(P.begin(), P.end(),compare);
        // Build lower hull
        for (int i = 0; i < n; ++i) {
                while (k >= 2 && cross(H[k-2], H[k-1], P[i]) <= 0) k--;
                H[k++] = P[i];
        }

        // Build upper hull
        //i starts from n-2 because n-1 is the point which both hulls will have in common
        //t=k+1 so that the upper hull has atleast two points to begin with
        for (int i = n-2, t = k+1; i >= 0; i--) {
                while (k >= t && cross(H[k-2], H[k-1], P[i]) <= 0) k--;
                H[k++] = P[i];
        }
        //the last point of upper hull is same with the fist point of the lower hull
        H.resize(k-1);
        return H;
}

$<br>$